Intersecting Chords Form A Pair Of Congruent Vertical Angles
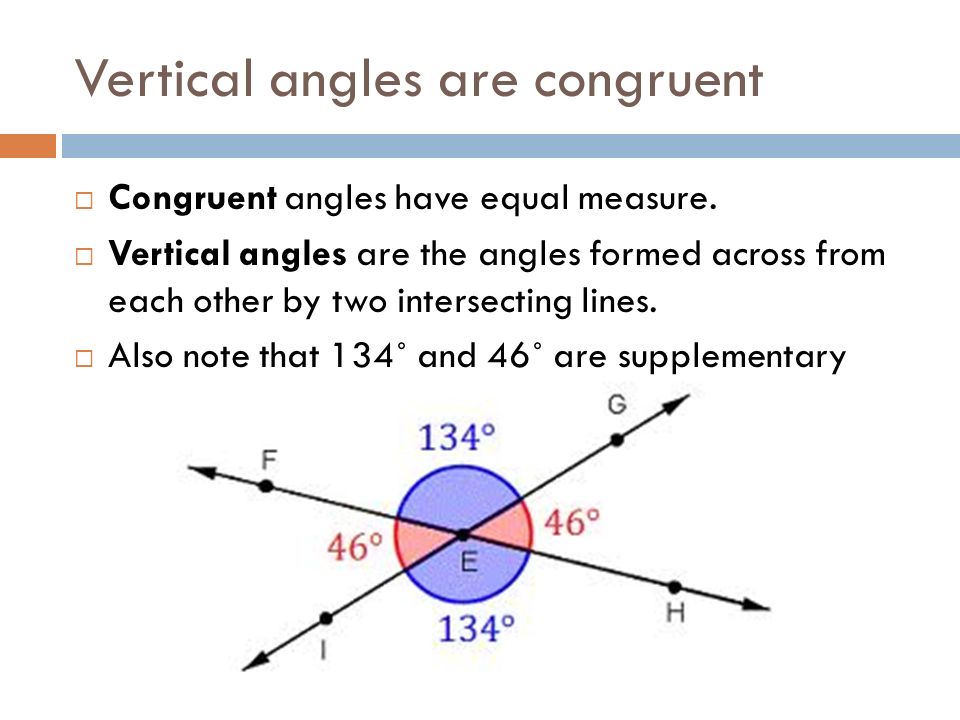
Intersecting Chords Form A Pair Of Congruent Vertical Angles - Web a simple extension of the inscribed angle theorem shows that the measure of the angle of intersecting chords in a circle is equal to half the sum of the measure of the two arcs that the angle and its opposite (or vertical) angle subtend on the circle's perimeter. That is, in the drawing above, m∠α = ½ (p+q). Web if two chords intersect inside a circle, then the measure of the angle formed is one half the sum of the measure of the arcs intercepted by the angle and its vertical angle. Additionally, the endpoints of the chords divide the circle into arcs. Vertical angles are formed and located opposite of each other having the same value. What happens when two chords intersect? According to the intersecting chords theorem, if two chords intersect inside a circle so that one is divided into segments of length \(a\) and \(b\) and the other into segments of length \(c\) and \(d\), then \(ab = cd\). In the circle, the two chords ¯ pr and ¯ qs intersect inside the circle. Since vertical angles are congruent, m∠1 = m∠3 and m∠2 = m∠4. A chord of a circle is a straight line segment whose endpoints both lie on the circle.
A chord of a circle is a straight line segment whose endpoints both lie on the circle. Not unless the chords are both diameters. Since vertical angles are congruent, m∠1 = m∠3 and m∠2 = m∠4. Web do intersecting chords form a pair of vertical angles? Vertical angles are formed and located opposite of each other having the same value. That is, in the drawing above, m∠α = ½ (p+q). Additionally, the endpoints of the chords divide the circle into arcs. If two chords intersect inside a circle, four angles are formed. Are two chords congruent if and only if the associated central. Thus, the answer to this item is true.
Intersecting chords form a pair of congruent vertical angles. Vertical angles are the angles opposite each other when two lines cross. In the diagram above, chords ab and cd intersect at p forming 2 pairs of congruent vertical angles, ∠apd≅∠cpb and ∠apc≅∠dpb. Not unless the chords are both diameters. Thus, the answer to this item is true. A chord of a circle is a straight line segment whose endpoints both lie on the circle. Web if two chords intersect inside a circle, then the measure of the angle formed is one half the sum of the measure of the arcs intercepted by the angle and its vertical angle. Web do intersecting chords form a pair of vertical angles? ∠2 and ∠4 are also a pair of vertical angles. Web i believe the answer to this item is the first choice, true.
Math 010 Chapter 9 Geometry Lines, figures, & triangles ppt video
According to the intersecting chords theorem, if two chords intersect inside a circle so that one is divided into segments of length \(a\) and \(b\) and the other into segments of length \(c\) and \(d\), then \(ab = cd\). That is, in the drawing above, m∠α = ½ (p+q). ∠2 and ∠4 are also a pair of vertical angles. Web.
Explore the properties of angles formed by two intersecting chords.1
Web if two chords intersect inside a circle, then the measure of the angle formed is one half the sum of the measure of the arcs intercepted by the angle and its vertical angle. How do you find the angle of intersecting chords? Web do intersecting chords form a pair of vertical angles? Web i believe the answer to this.
How to Prove the Intersecting Chords Theorem of Euclid 7 Steps
I believe the answer to this item is the first choice, true. If two chords intersect inside a circle, four angles are formed. That is, in the drawing above, m∠α = ½ (p+q). Are two chords congruent if and only if the associated central. ∠2 and ∠4 are also a pair of vertical angles.
Vertical Angles Cuemath
Web a simple extension of the inscribed angle theorem shows that the measure of the angle of intersecting chords in a circle is equal to half the sum of the measure of the two arcs that the angle and its opposite (or vertical) angle subtend on the circle's perimeter. Vertical angles are formed and located opposite of each other having.
Intersecting Chords Form A Pair Of Congruent Vertical Angles
Intersecting chords form a pair of congruent vertical angles. Any intersecting segments (chords or not) form a pair of congruent, vertical angles. ∠2 and ∠4 are also a pair of vertical angles. Intersecting chords form a pair of congruent vertical angles. Additionally, the endpoints of the chords divide the circle into arcs.
Intersecting Chords Form A Pair Of Supplementary Vertical Angles
Web if two chords intersect inside a circle, then the measure of the angle formed is one half the sum of the measure of the arcs intercepted by the angle and its vertical angle. Not unless the chords are both diameters. Intersecting chords form a pair of congruent vertical angles. In the circle, the two chords ¯ pr and ¯.
Explore the properties of angles formed by two intersecting chords. 1
Additionally, the endpoints of the chords divide the circle into arcs. I believe the answer to this item is the first choice, true. Web a simple extension of the inscribed angle theorem shows that the measure of the angle of intersecting chords in a circle is equal to half the sum of the measure of the two arcs that the.
When chords intersect in a circle, the vertical angles formed intercept
That is, in the drawing above, m∠α = ½ (p+q). Web do intersecting chords form a pair of vertical angles? A chord of a circle is a straight line segment whose endpoints both lie on the circle. Any intersecting segments (chords or not) form a pair of congruent, vertical angles. Intersecting chords form a pair of congruent vertical angles.
Intersecting Chords Form A Pair Of Supplementary Vertical Angles
Web if two chords intersect inside a circle, then the measure of the angle formed is one half the sum of the measure of the arcs intercepted by the angle and its vertical angle. ∠2 and ∠4 are also a pair of vertical angles. Web intersecting chords theorem: Web do intersecting chords form a pair of vertical angles? Web i.
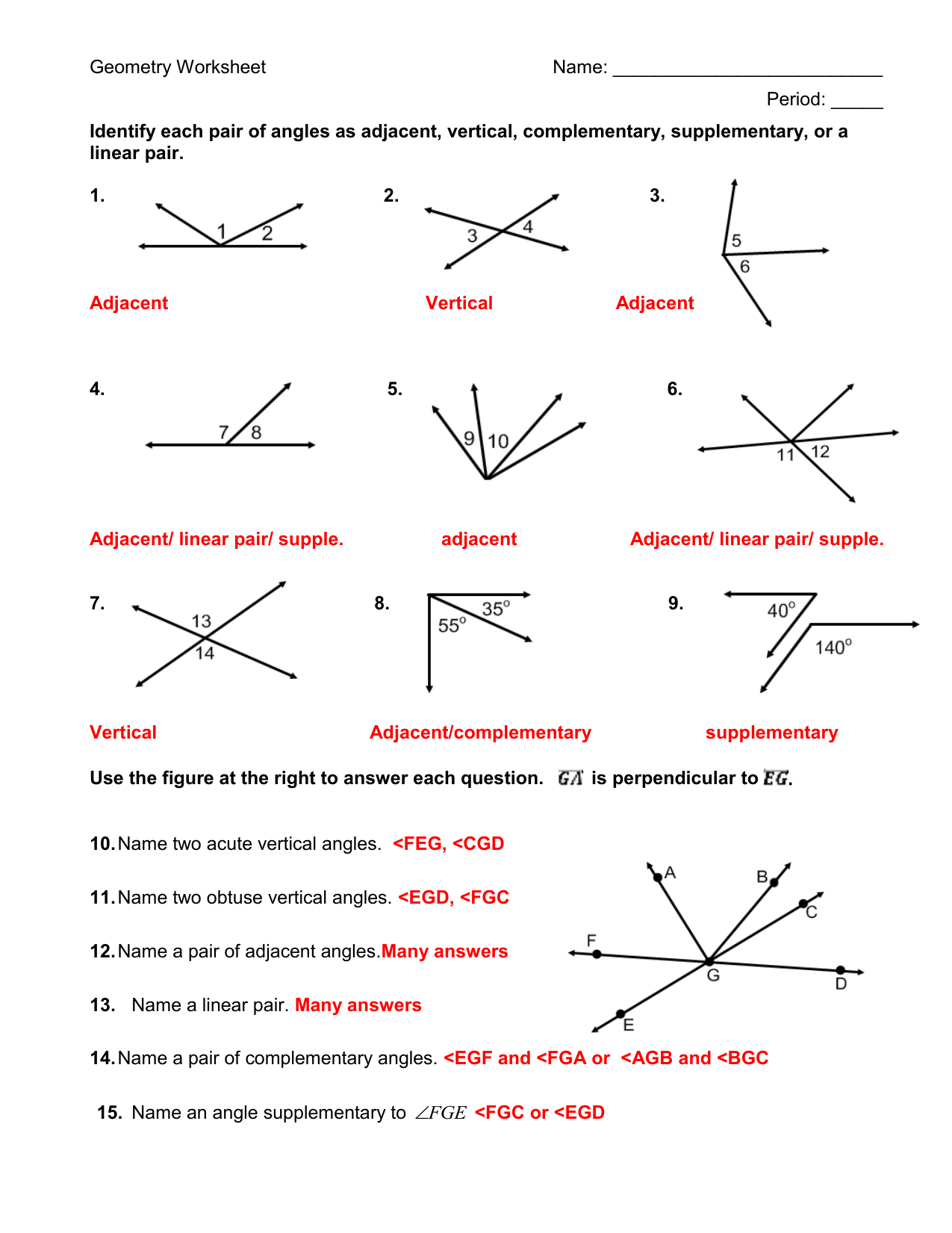
Pairs Of Angles Worksheet Answers —
What happens when two chords intersect? Any intersecting segments (chords or not) form a pair of congruent, vertical angles. Thus, the answer to this item is true. Since vertical angles are congruent, m∠1 = m∠3 and m∠2 = m∠4. I believe the answer to this item is the first choice, true.
How Do You Find The Angle Of Intersecting Chords?
Web i believe the answer to this item is the first choice, true. Any intersecting segments (chords or not) form a pair of congruent, vertical angles. Are two chords congruent if and only if the associated central. Web do intersecting chords form a pair of vertical angles?
In The Diagram Above, ∠1 And ∠3 Are A Pair Of Vertical Angles.
∠2 and ∠4 are also a pair of vertical angles. Since vertical angles are congruent, m∠1 = m∠3 and m∠2 = m∠4. Vertical angles are formed and located opposite of each other having the same value. A chord of a circle is a straight line segment whose endpoints both lie on the circle.
I Believe The Answer To This Item Is The First Choice, True.
In the diagram above, chords ab and cd intersect at p forming 2 pairs of congruent vertical angles, ∠apd≅∠cpb and ∠apc≅∠dpb. Intersecting chords form a pair of congruent vertical angles. That is, in the drawing above, m∠α = ½ (p+q). Thus, the answer to this item is true.
Not Unless The Chords Are Both Diameters.
Web if two chords intersect inside a circle, then the measure of the angle formed is one half the sum of the measure of the arcs intercepted by the angle and its vertical angle. Web when chords intersect in a circle are the vertical angles formed intercept congruent arcs? Vertical angles are the angles opposite each other when two lines cross. In the circle, the two chords ¯ pr and ¯ qs intersect inside the circle.