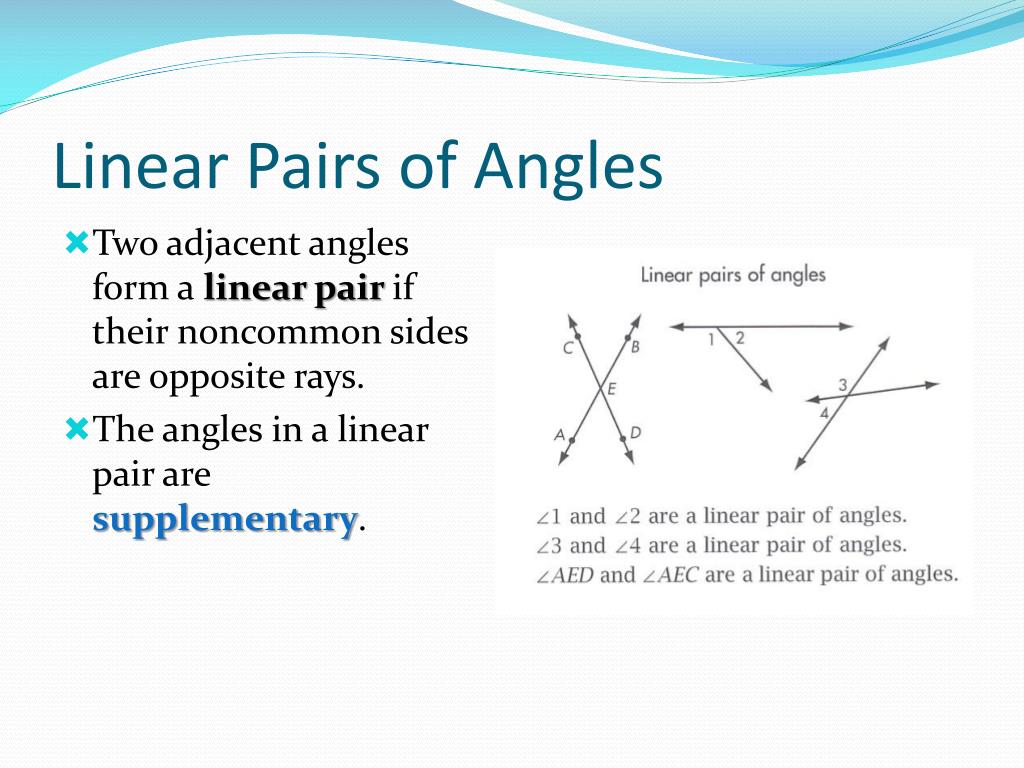
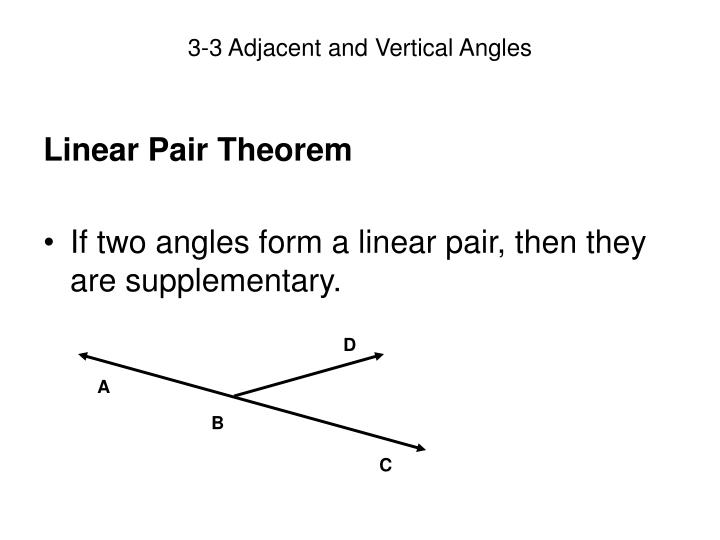
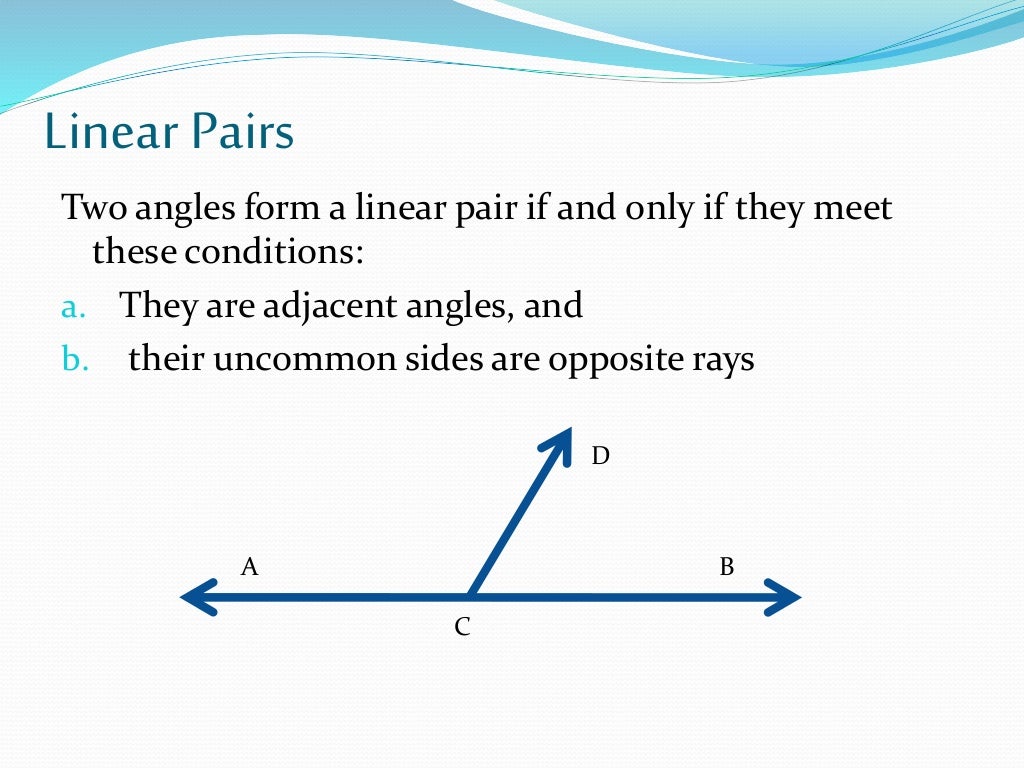
Two Angles That Form A Linear Pair
Two Angles That Form A Linear Pair - We now have an equation in two unknowns. Web up to 6% cash back the supplement postulate states that if two angles form a linear pair , then they are supplementary. If the two angles form a linear pair, then the sum of the two angles equals 180 degrees. This fact leads to a wide range of properties and applications. The sum of linear pairs is 180°. Since the sum of angles is not equal to 90 °, the angles 50 ° and 40 ° do. In the figure, ∠ 1 and ∠ 2 form a linear pair. Linear pairs of angles are also referred to as supplementary. Web first we need to define what is a linear pair? In the figure, ∠ 1 and ∠ 2 are supplementary by the.
So do ∠ 2 and ∠ 3 , ∠ 3 and ∠ 4 , and. Web not all supplementary angle form a linear pair. The sum of linear pairs is 180°. We now have an equation in two unknowns. This fact leads to a wide range of properties and applications. In the figure, ∠ 1 and ∠ 2 are supplementary by the. Web up to 6% cash back the supplement postulate states that if two angles form a linear pair , then they are supplementary. Web linear pair of angles are two angles that form a straight angle (angle measuring 180 degrees). If the two angles form a linear pair, then the sum of the two angles equals 180 degrees. Since the sum of angles is not equal to 90 °, the angles 50 ° and 40 ° do.
Web not all supplementary angle form a linear pair. In the given diagram, o a and o b are. It should be noted that all linear pairs are supplementary because. In the diagram below, ∠abc and ∠dbe are supplementary since 30°+150°=180°,. Web however, just because two angles are supplementary does not mean they form a linear pair. Since the sum of angles is not equal to 90 °, the angles 50 ° and 40 ° do. Supplementary angles are two angles whose same is 180^o linear. In the figure, ∠ 1 and ∠ 2 form a linear pair. This fact leads to a wide range of properties and applications. (a) 50 ° + 40 ° = 90 °.
Two angles form a linear pair. The measure of one CameraMath
A linear pair are two angles that makes a line. In the given diagram, o a and o b are. (a) 50 ° + 40 ° = 90 °. Two angles are said to form a linear pair if they add up to 180 degrees. We now have an equation in two unknowns.
PPT Geometry Review Angles and Parallel Lines PowerPoint Presentation
This fact leads to a wide range of properties and applications. In the figure, ∠ 1 and ∠ 2 are supplementary by the. Web not all supplementary angle form a linear pair. In the given diagram, o a and o b are. Since the sum of angles is not equal to 90 °, the angles 50 ° and 40 °.
Which statement is true about this argument? Premises If two angles
In the figure, ∠ 1 and ∠ 2 are supplementary by the. But, all linear pairs are supplementary. Web first we need to define what is a linear pair? Web when two lines intersect each other, the adjacent angles make a linear pair. Linear pairs of angles are also referred to as supplementary.
PPT 33 Adjacent and Vertical Angles PowerPoint Presentation ID6953038
In the diagram below, ∠abc and ∠dbe are supplementary since 30°+150°=180°,. Web the linear pair postulate says if two angles form a linear pair, then the measures of the angles add up to 180°. Web up to 6% cash back the supplement postulate states that if two angles form a linear pair , then they are supplementary. Two angles are.
Definition and Examples of Linear Pairs YouTube
This fact leads to a wide range of properties and applications. The sum of two angles in the linear pair is always 180 degrees. It should be noted that all linear pairs are supplementary because. A linear pair are two angles that makes a line. Two angles are said to form a linear pair if they add up to 180.
📈In which diagram do angles 1 and 2 form a linear pair?
If the two angles form a linear pair, then the sum of the two angles equals 180 degrees. Web up to 6% cash back a linear pair is a pair of adjacent angles formed when two lines intersect. So do ∠ 2 and ∠ 3 , ∠ 3 and ∠ 4 , and. Web the two angles make a linear.
Linear pair
Web up to 6% cash back the supplement postulate states that if two angles form a linear pair , then they are supplementary. Web linear pair of angles are two angles that form a straight angle (angle measuring 180 degrees). (a) 50 ° + 40 ° = 90 °. In the figure, ∠ 1 and ∠ 2 are supplementary by.
The two angles below form a linear pair, and the expressions are
We now have an equation in two unknowns. In the figure, ∠ 1 and ∠ 2 form a linear pair. Web when two lines intersect each other, the adjacent angles make a linear pair. But, all linear pairs are supplementary. So that means <1 + <2 =180 but let’s call those.
Name two angles that form a linear pair.
The sum of linear pairs is 180°. Linear pairs of angles are also referred to as supplementary. A linear pair are two angles that makes a line. In the figure, ∠ 1 and ∠ 2 are supplementary by the. In the given diagram, o a and o b are.
Linear Pair lines and angles This postulate is sometimes call the
But, all linear pairs are supplementary. Since the sum of angles is not equal to 90 °, the angles 50 ° and 40 ° do. Web up to 6% cash back a linear pair is a pair of adjacent angles formed when two lines intersect. Web not all supplementary angle form a linear pair. Web up to 6% cash back.
Linear Pairs Of Angles Are Also Referred To As Supplementary.
Web however, just because two angles are supplementary does not mean they form a linear pair. The sum of two angles in the linear pair is always 180 degrees. So that means <1 + <2 =180 but let’s call those. A line is 180 degrees.
(A) 50 ° + 40 ° = 90 °.
In the diagram below, ∠abc and ∠dbe are supplementary since 30°+150°=180°,. The sum of linear pairs is 180°. Two angles are said to form a linear pair if they add up to 180 degrees. But, all linear pairs are supplementary.
If The Two Angles Form A Linear Pair, Then The Sum Of The Two Angles Equals 180 Degrees.
Web the linear pair postulate says if two angles form a linear pair, then the measures of the angles add up to 180°. We now have an equation in two unknowns. In the given diagram, o a and o b are. The steps to using this postulate are very.
This Fact Leads To A Wide Range Of Properties And Applications.
Web the two angles make a linear pair, so the sum of measures of the two angles is 180°\text{\textdegree}°. Web not all supplementary angle form a linear pair. Web first we need to define what is a linear pair? Web when two lines intersect each other, the adjacent angles make a linear pair.