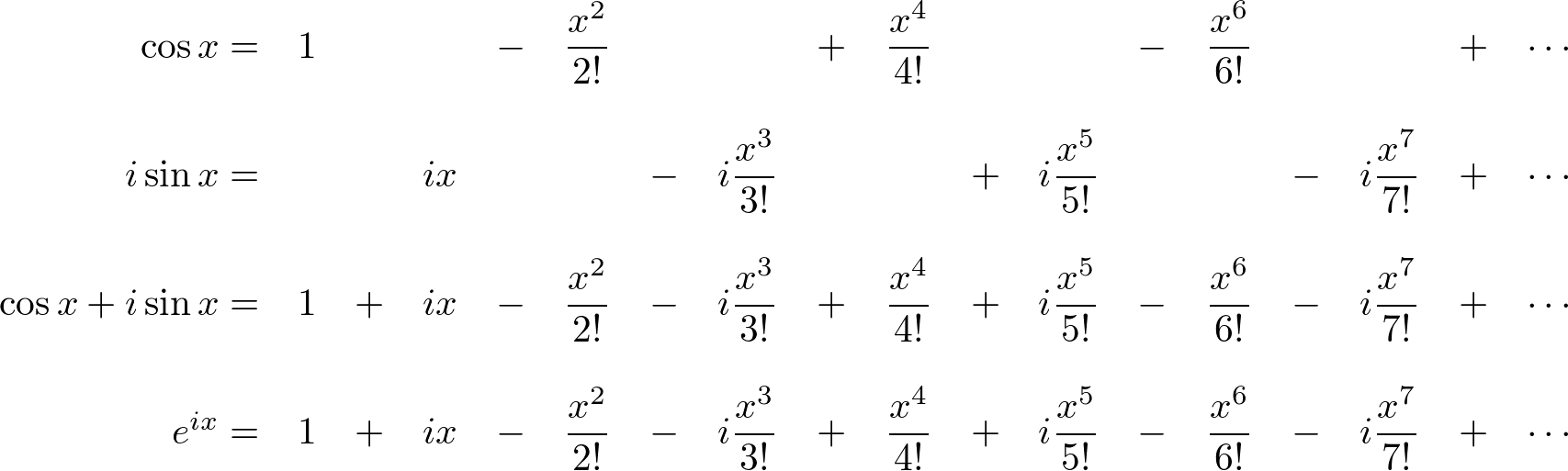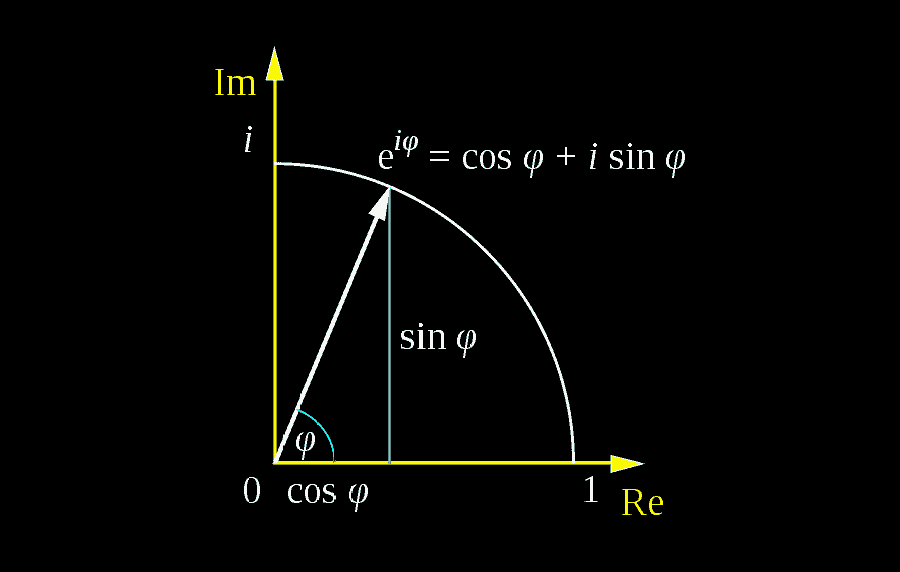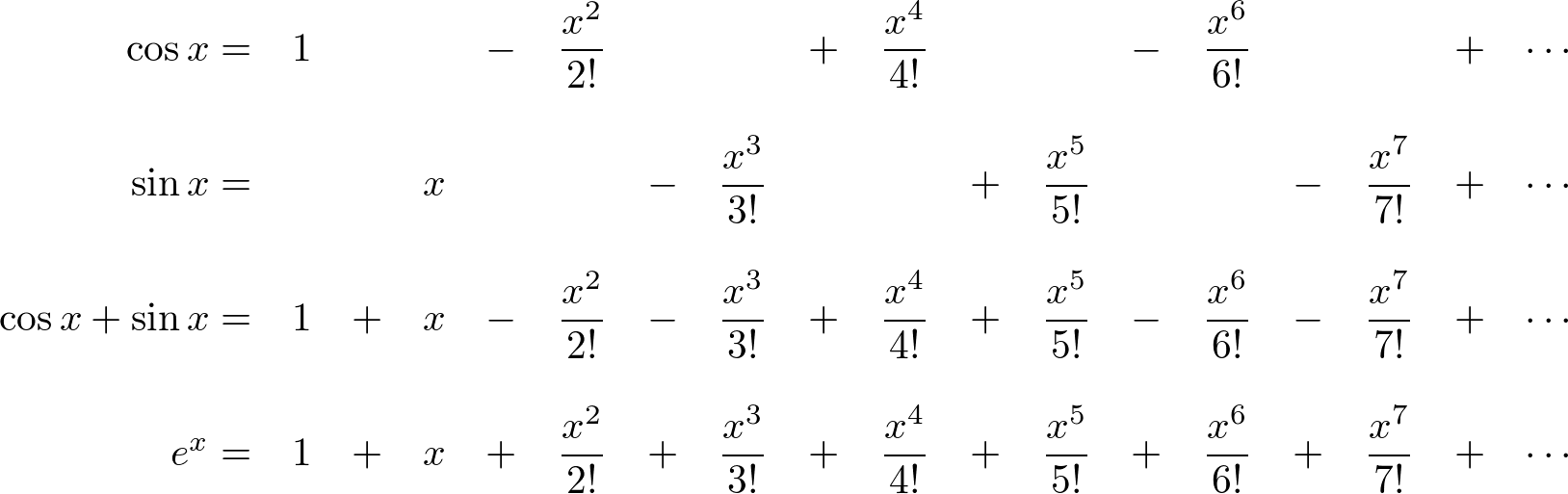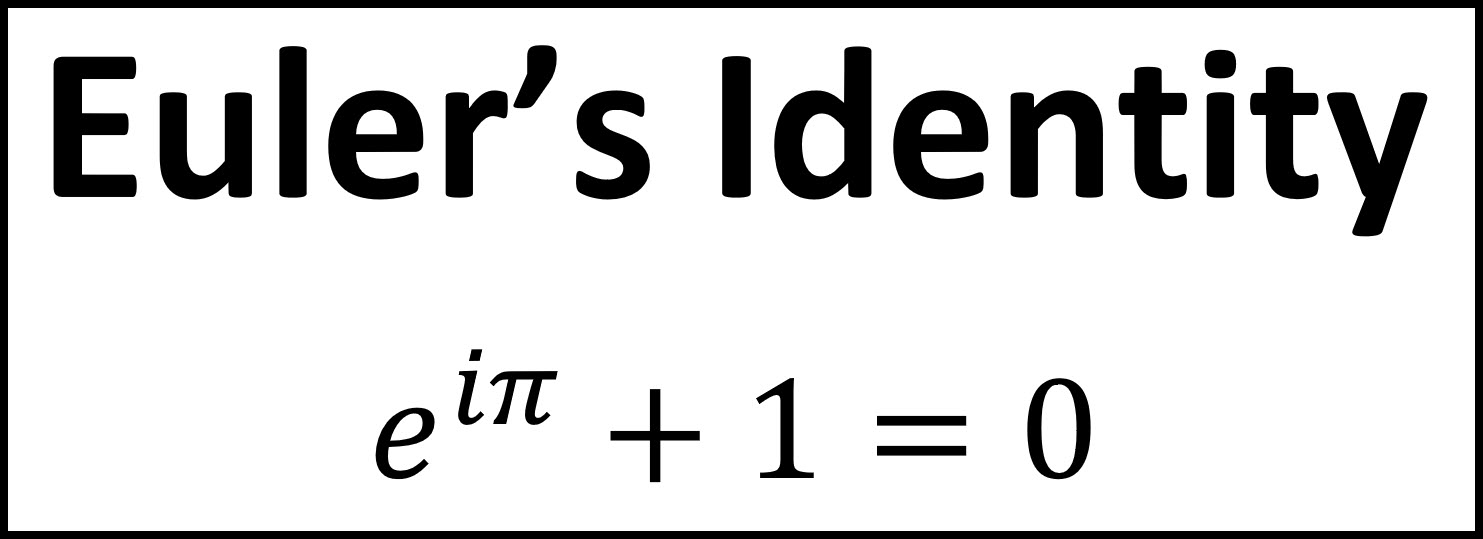Sin In Euler Form
Sin In Euler Form - There are many ways to approach euler’s formula. −i = i sin(3π/2) 1 + i = √ 2(cos(π/4)+ i sin(π/4)) −1 + i √ 3 = 2(cos(2π/3)+ i sin(2π/3)) 1 − i = √ 2(cos(−π/4)+ i sin(−π/4)). Using the formula derived above, we plug 3 + 4i in for θ: Web euler’sproductformulafor sineforany complexnumberz, sin τ 2 z = τ 2 z 1 − z2 12! Web euler and the function sin(x)/x in the early 17 hundreds the great swiss mathematician leonard euler working alternatively at the russian and the prussian. Web find sin (3 + 4i) using euler's formula: Let be an angle measured. The pink spinning number is. Our approach is to simply take equation 1.6.1 as the definition. Web leonhard euler's introductio in analysin infinitorum (1748) was mostly responsible for establishing the analytic treatment of trigonometric functions in europe, also defining.
Using the formula derived above, we plug 3 + 4i in for θ: Sinn und form ( german: −i = i sin(3π/2) 1 + i = √ 2(cos(π/4)+ i sin(π/4)) −1 + i √ 3 = 2(cos(2π/3)+ i sin(2π/3)) 1 − i = √ 2(cos(−π/4)+ i sin(−π/4)). Euler’s formula, at least for real values and glossing over several. Web euler’sproductformulafor sineforany complexnumberz, sin τ 2 z = τ 2 z 1 − z2 12! There are many ways to approach euler’s formula. Web the absolute position is transmitted in the form of a digital ssi or biss data word. Web euler formula and sin 3 ask question asked 12 years ago modified 11 years, 8 months ago viewed 8k times 3 using the formula: Sin ( x + y) =. In this lesson we will explore the derivation of several trigonometric identities, namely.
There are many ways to approach euler’s formula. E i ω t = cos ω t + i sin ω t i would like to prove. Sense and form) is a bimonthly literary and culture magazine. These representations can be used to prove many trigonometric. Web euler’sproductformulafor sineforany complexnumberz, sin τ 2 z = τ 2 z 1 − z2 12! Sin ( x + y) =. Web one might imagine proving that euler’s infinite product equals sin(πz) by taking the logarithm of both sides — we need only show that log(sin(πz)) = log(πz) + x∞ n=1 log 1. Web the formula is the following: In this lesson we will explore the derivation of several trigonometric identities, namely. The pink spinning number is.
Euler's Equation
In this lesson we will explore the derivation of several trigonometric identities, namely. Web euler's identity is a special case of euler's formula, which states that for any real number x, e i x = cos x + i sin x {\displaystyle e^{ix}=\cos x+i\sin x} where the inputs of the. −i = i sin(3π/2) 1 + i =.
Desmos A Definitive Guide on Graphing and Computing Math Vault
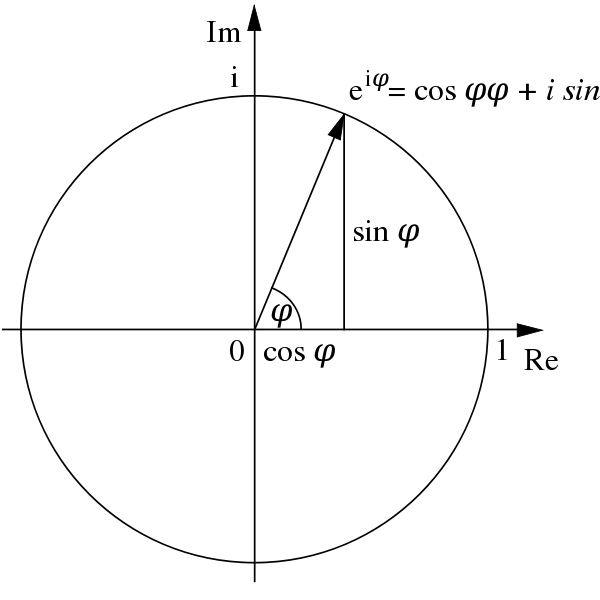
Web euler’sproductformulafor sineforany complexnumberz, sin τ 2 z = τ 2 z 1 − z2 12! Hence, find the solutions of s i n s i n 5 𝜃 − 3 𝜃 = 0 in the. −i,1 + i,1 − i, −1 + i √ 3. It tells us that e raised to any imaginary number will produce a point.
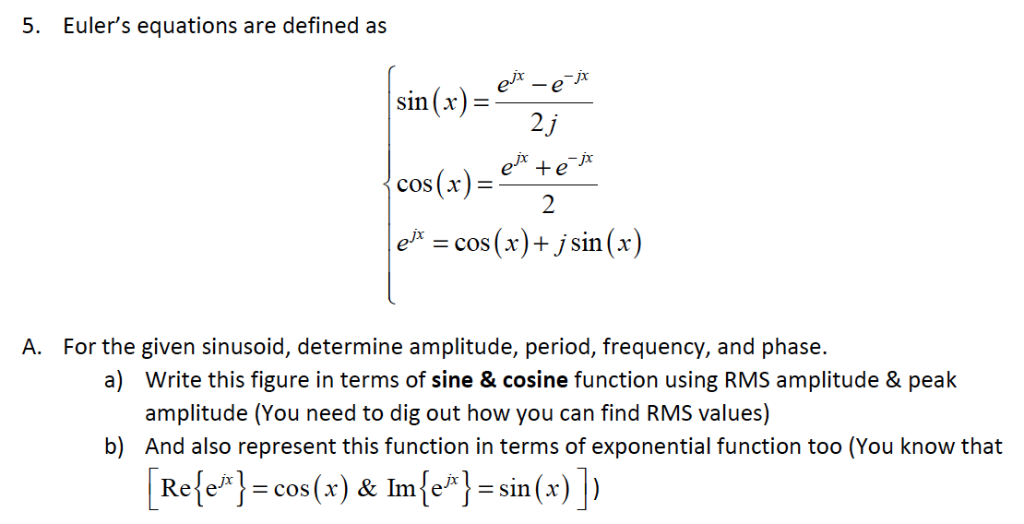
Solved 5. Euler's equations are defined as sin (x) cos(x) e"
Web euler’sproductformulafor sineforany complexnumberz, sin τ 2 z = τ 2 z 1 − z2 12! = = from euler's formula, plugging these into the formula for sin (3 + 4i) yields: Web find sin (3 + 4i) using euler's formula: It tells us that e raised to any imaginary number will produce a point on the unit. Euler’s formula,.
FileEuler's formula.svg New World Encyclopedia
Web find sin (3 + 4i) using euler's formula: It was launched in east berlin, east germany, in. Web the sine function is one of the basic functions encountered in trigonometry (the others being the cosecant, cosine , cotangent, secant, and tangent ). Hence, find the solutions of s i n s i n 5 𝜃 − 3 𝜃 =.
Eulers sine wave YouTube
Web euler's identity is a special case of euler's formula, which states that for any real number x, e i x = cos x + i sin x {\displaystyle e^{ix}=\cos x+i\sin x} where the inputs of the. Web euler’sproductformulafor sineforany complexnumberz, sin τ 2 z = τ 2 z 1 − z2 12! The pink spinning number is..
HMI Update for day Euler's Equation
Web euler and the function sin(x)/x in the early 17 hundreds the great swiss mathematician leonard euler working alternatively at the russian and the prussian. Web give the polar form for: = = from euler's formula, plugging these into the formula for sin (3 + 4i) yields: Web euler formula and sin 3 ask question asked 12 years ago modified.
Euler's Formula YouTube
Sense and form) is a bimonthly literary and culture magazine. Web euler's identity is a special case of euler's formula, which states that for any real number x, e i x = cos x + i sin x {\displaystyle e^{ix}=\cos x+i\sin x} where the inputs of the. Web euler and the function sin(x)/x in the early 17 hundreds.
Deriving the famous Euler’s formula through Taylor Series Muthukrishnan
The pink spinning number is. Web euler’sproductformulafor sineforany complexnumberz, sin τ 2 z = τ 2 z 1 − z2 12! Sin ( x + y) =. Web one might imagine proving that euler’s infinite product equals sin(πz) by taking the logarithm of both sides — we need only show that log(sin(πz)) = log(πz) + x∞ n=1 log 1. =.
LEONHARD EULER Biografía, Aportes, Obras, Frases, y más
Hence, find the solutions of s i n s i n 5 𝜃 − 3 𝜃 = 0 in the. Web use euler’s formula to express s i n c o s 𝜃 𝜃 in the form 𝑎 𝜃 + 𝑏 3 𝜃 + 𝑐 5 𝜃 s i n s i n s i n, where 𝑎, 𝑏, and.
Euler's Formula
There are many ways to approach euler’s formula. Web the formula is the following: Web leonhard euler's introductio in analysin infinitorum (1748) was mostly responsible for establishing the analytic treatment of trigonometric functions in europe, also defining. Web find sin (3 + 4i) using euler's formula: Web euler’sproductformulafor sineforany complexnumberz, sin τ 2 z = τ 2 z 1 −.
Web Euler’s Formula For Complex Exponentials According To Euler, We Should Regard The Complex Exponential Eit As Related To The Trigonometric Functions Cos(T) And.
It tells us that e raised to any imaginary number will produce a point on the unit. Web give the polar form for: Using the formula derived above, we plug 3 + 4i in for θ: Web the formula is the following:
Our Approach Is To Simply Take Equation 1.6.1 As The Definition.
Web the absolute position is transmitted in the form of a digital ssi or biss data word. −i = i sin(3π/2) 1 + i = √ 2(cos(π/4)+ i sin(π/4)) −1 + i √ 3 = 2(cos(2π/3)+ i sin(2π/3)) 1 − i = √ 2(cos(−π/4)+ i sin(−π/4)). Web one might imagine proving that euler’s infinite product equals sin(πz) by taking the logarithm of both sides — we need only show that log(sin(πz)) = log(πz) + x∞ n=1 log 1. Web find sin (3 + 4i) using euler's formula:
Let Be An Angle Measured.
Sinn und form ( german: Euler’s formula, at least for real values and glossing over several. Web euler and the function sin(x)/x in the early 17 hundreds the great swiss mathematician leonard euler working alternatively at the russian and the prussian. Web euler formula and sin 3 ask question asked 12 years ago modified 11 years, 8 months ago viewed 8k times 3 using the formula:
Web Euler’sproductformulafor Sineforany Complexnumberz, Sin Τ 2 Z = Τ 2 Z 1 − Z2 12!
Web euler's formula is used to express the sine and cosine functions as a sum of complex exponentials. These representations can be used to prove many trigonometric. −i,1 + i,1 − i, −1 + i √ 3. Hence, find the solutions of s i n s i n 5 𝜃 − 3 𝜃 = 0 in the.