Parametric Equations In Rectangular Form

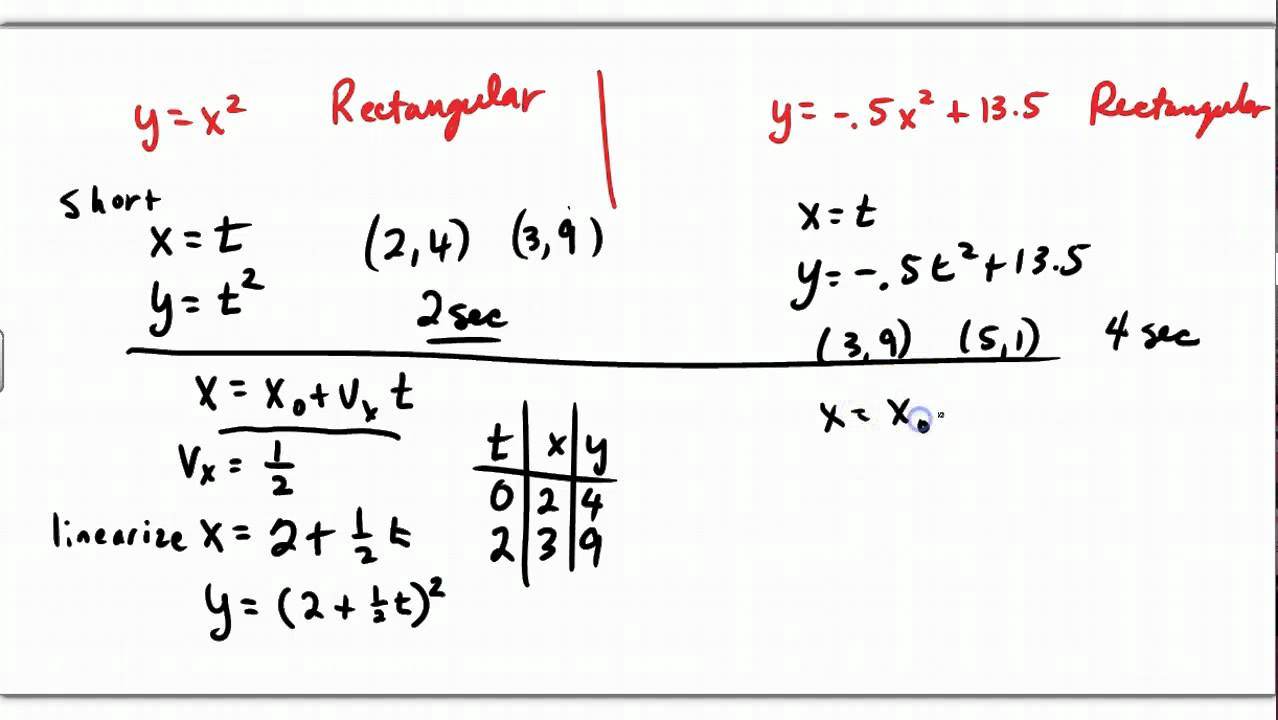
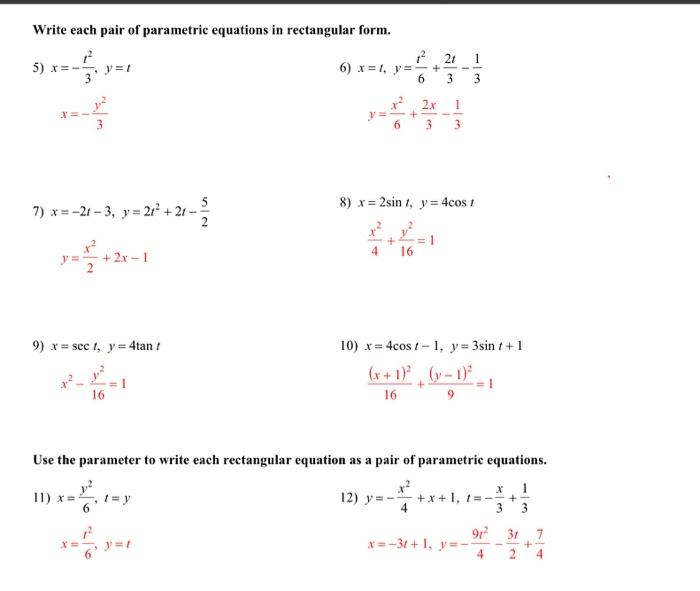
Parametric Equations In Rectangular Form - Convert to rectangular x=t^2 , y=t^9. Web together, x(t) and y(t) are called parametric equations, and generate an ordered pair (x(t), y(t)). Rewrite the equation as t2 = x t 2 = x. Converting from rectangular to parametric can be very simple: 4.2k views 2 years ago parametric equations. Write the parametric equations in rectangular form and identify the interval for x or y line example show more. Web converting parametric equation to a cartesian equation or rectangular form involves solving for t in terms of x and then plugging this into the y equation. Web convert the parametric equations 𝑥 equals 𝑡 squared plus two and 𝑦 equals three 𝑡 minus one to rectangular form. You have to solve for \(t\) in one of the equations. T2 = x t 2 = x.

F (t)= (x (t),y (t)) given x (t)=t,y (t)=3−t2 select one: For example y = 4 x + 3 is a rectangular equation. Set up the parametric equation for x(t) x ( t) to solve the equation for t t. X = t2 x = t 2 , y = t9 y = t 9. Web converting parametric equation to rectangular form. Web writing parametric equations in rectangular form Given \(y=f(x)\), the parametric equations \(x=t\), \(y=f(t)\) produce the same graph. To convert parametric equations to rectangular form, we need to find a way to eliminate the 𝑡. Web convert the parametric equations 𝑥 equals 𝑡 squared plus two and 𝑦 equals three 𝑡 minus one to rectangular form. Y = (x+3)^2 + 5.

Eliminate the parameter from the parametric equations below, and convert the equation into rectangular form. Y = 3x3 + 5x +6. Y = 3x 3 + 5x +6; Web convert the parametric equations 𝑥 is equal to the cos of 𝑡 and 𝑦 is equal to the sin of 𝑡 to rectangular form. By convention, t is frequently used as the parameter, though other variables can be used as well. From the curve’s vertex at (1, 2), the graph sweeps out to the right. Web write down the following mentioned rectangular equations into parametric form. Web together, x(t) and y(t) are called parametric equations, and generate an ordered pair (x(t), y(t)). Web parametric equations are a set of equations that express a set of quantities as explicit functions of a number of independent variables, known as parameters. for example, while the equation of a circle in cartesian coordinates can be given by r^2=x^2+y^2, one set of parametric equations for the circle are given by x = rcost (1) y. Although we have just shown that there is only one way to interpret a set of parametric equations as a rectangular equation, there are multiple ways to interpret a rectangular equation as a set of parametric equations.

Parametric Equations Rectangular Form YouTube

Sometimes the parametric equations for the individual scalar output variables are combined into a single parametric equation in vectors : For parametric equations, put x = t so, the equation becomes, y = 3t 3 + 5t + 6 By convention, t is frequently used as the parameter, though other variables can be used as well. Web convert the parametric.

Rectangular Form Of Parametric Equations akrisztina27

Web convert the parametric equations 𝑥 equals 𝑡 squared plus two and 𝑦 equals three 𝑡 minus one to rectangular form. Y = 3x 3 + 5x +6; Web converting parametric equations to rectangular: For parametric equations, put x = t so, the equation becomes, y = 3t 3 + 5t + 6 Converting from rectangular to parametric can be.

Precalculus Chapter 6.3 Exercises 1118 Convert Parametric Equations

Here, we have a pair of parametric equations. T2 = x t 2 = x. For parametric equations, put x = t so, the equation becomes, y = 3t 3 + 5t + 6 A point ( x, y) is on the unit circle if and only if there is a value of t such that these two equations generate.

Rectangular Form Of Parametric Equations akrisztina27

To convert parametric equations to rectangular form, we need to find a way to eliminate the 𝑡. Web example 1 sketching the graph of a pair of parametric equations by plotting points sketch the graph of the parametric equations x(t) = t2 + 1, y(t) = 2 + t. F (t)= (x (t),y (t)) given x (t)=t,y (t)=3−t2 select one:.

Rectangular Form Of Parametric Equations akrisztina27

Eliminate the parameter from the parametric equations below, and convert the equation into rectangular form. Y = 3x 3 + 5x +6; When we parameterize a curve, we are translating a single equation in two variables, such as x and y ,into an equivalent pair of equations in three variables, x, y, and t. Web convert the parametric equations 𝑥.

Rectangular Form Of Parametric Equations akrisztina27

You have to solve for \(t\) in one of the equations. For example y = 4 x + 3 is a rectangular equation. Given \(y=f(x)\), the parametric equations \(x=t\), \(y=f(t)\) produce the same graph. Web 1 day agoyou'll get a detailed solution from a subject matter expert that helps you learn core concepts. Web convert x^2 + y^2 = 1.

How to convert parametric equations to rectangular form example 3 YouTube

To convert this rectangular equation to parametric form, we make use of our knowledge of trigonometry and its identities. X = t2 x = t 2. Web convert the parametric equations 𝑥 equals 𝑡 squared plus two and 𝑦 equals three 𝑡 minus one to rectangular form. We want to eliminate our. Y = 3x3 + 5x +6.

Rectangular Form Of Parametric Equations akrisztina27

We want to eliminate our. Web converting parametric equations to rectangular: Web converting between rectangular and parametric equations. Set up the parametric equation for x(t) x ( t) to solve the equation for t t. F (t)= (x (t),y (t)) given x (t)=t,y (t)=3−t2 select one:

SOLVEDFind a rectangular equation equivalent to the given pair of

By convention, t is frequently used as the parameter, though other variables can be used as well. X = t2 x = t 2. Web form a parametric representation of the unit circle, where t is the parameter: Web convert the parametric equations 𝑥 is equal to the cos of 𝑡 and 𝑦 is equal to the sin of 𝑡.

Rectangular Form Of Parametric Equations akrisztina27

Converting from rectangular to parametric can be very simple: We have 𝑥 is equal to some function of 𝑡 and 𝑦 is equal to some other function of 𝑡. Web together, x(t) and y(t) are called parametric equations, and generate an ordered pair (x(t), y(t)). To convert parametric equations to rectangular form, we need to find a way to eliminate.

Web For The Following Exercises, Convert The Parametric Equations Of A Curve Into Rectangular Form.

F (t)= (x (t),y (t)) given x (t)=t,y (t)=3−t2 select one: Web find parametric equations for curves defined by rectangular equations. Y = x 4 + 5x 2 +8; Web 1 day agoyou'll get a detailed solution from a subject matter expert that helps you learn core concepts.

Convert To Rectangular X=T^2 , Y=T^9.

Although we have just shown that there is only one way to interpret a set of parametric equations as a rectangular equation, there are multiple ways to interpret a rectangular equation as a set of parametric equations. Web together, x(t) and y(t) are called parametric equations, and generate an ordered pair (x(t), y(t)). To convert parametric equations to rectangular form, we need to find a way to eliminate the 𝑡. Web in the rectangular coordinate system, the rectangular equation y = f ( x) works well for some shapes like a parabola with a vertical axis of symmetry, but in precalculus and the review of conic sections in section 10.0, we encountered several shapes that could not be sketched in this manner.

X = T2 X = T 2.

Web converting parametric equation to a cartesian equation or rectangular form involves solving for t in terms of x and then plugging this into the y equation. We have 𝑥 is equal to some function of 𝑡 and 𝑦 is equal to some other function of 𝑡. Web writing parametric equations in rectangular form Web a typical parametric equation will be in the form x = f ( t) and y = g ( t).

Web Converting Parametric Equations To Rectangular:

We’re given a pair of parametric equations, and we’re asked to convert this into the rectangular form. Web converting between rectangular and parametric equations. You have to solve for \(t\) in one of the equations. Web write down the following mentioned rectangular equations into parametric form.