How To Do Row Echelon Form
How To Do Row Echelon Form - All zero rows are at the bottom of the matrix. Web definition (row echelon form) a matrix m is said to be in row echelon form (ref) iff: Learn how the elimination method corresponds to performing row operations on an augmented. Now, we reduce the above matrix to row. Web what does row echelon form mean? Web learn to replace a system of linear equations by an augmented matrix. Elementary linear algebra (fourth edition), 2010. Below are a few examples of matrices in row echelon form: Information and translations of row echelon form in the most comprehensive dictionary definitions resource on the web. Web solution the correct answer is (b), since it satisfies all of the requirements for a row echelon matrix.
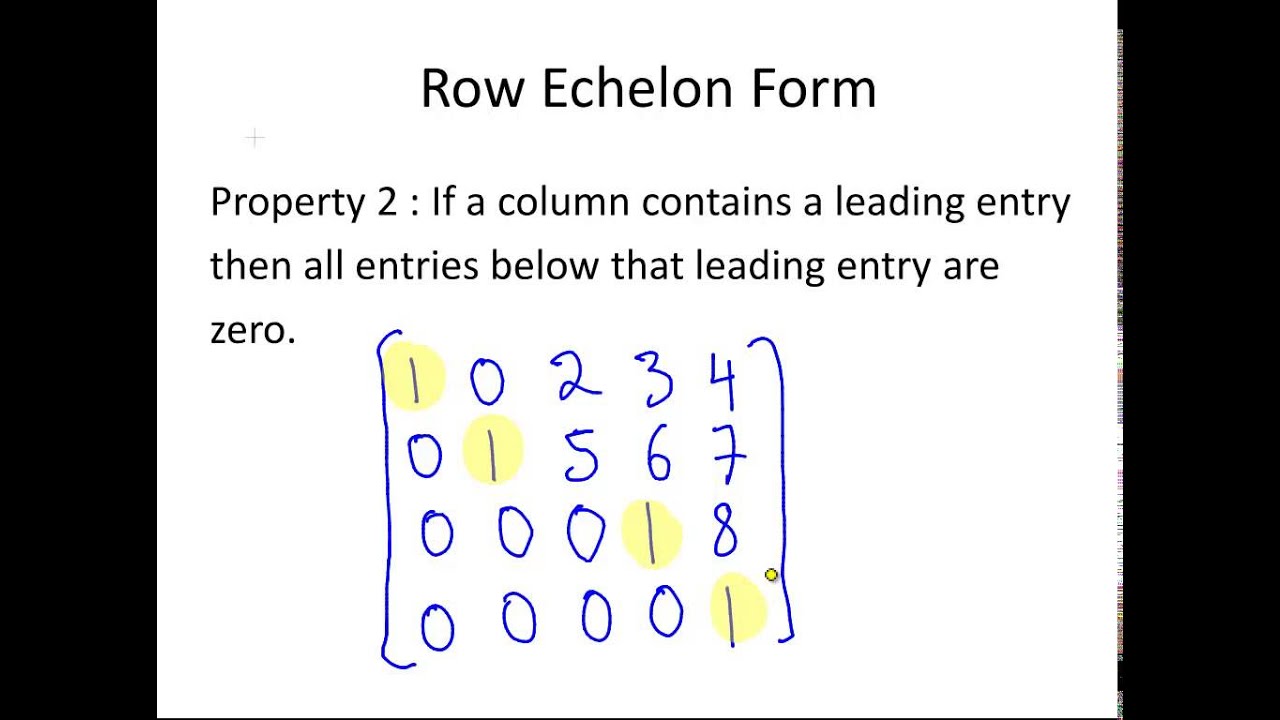
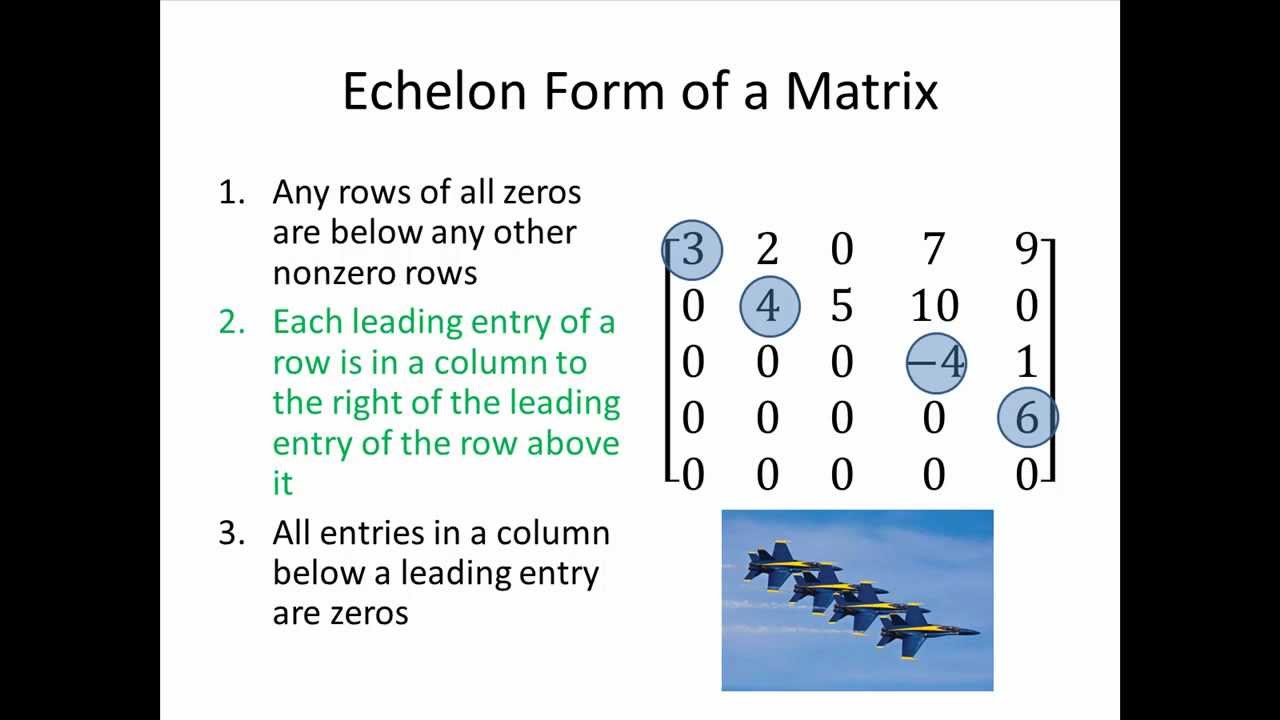
All nonzero rows are above all rows of zeros. You might want to use.echelon_form() instead. Web about press copyright contact us creators advertise developers terms privacy policy & safety how youtube works test new features press copyright contact us creators. Web what does row echelon form mean? Let’s take an example matrix: The leading entry of each nonzero row after the first occurs to the right of the leading entry of the previous row. Learn how the elimination method corresponds to performing row operations on an augmented. Web 1) 1) if you see a row which is a linear combination of the other rows then you can immediately replace it with a row of zeroes. Rows consisting of all zeros are at the bottom of the matrix. The leading entry in row 1 of matrix a is to the right.
All zero rows are at the bottom of the matrix. Technically, the leading coefficient can. Web about press copyright contact us creators advertise developers terms privacy policy & safety how youtube works test new features press copyright contact us creators. The leading entry of each nonzero row after the first occurs to the right of the leading entry of the previous row. Web instead of gaussian elimination and back substitution, a system of equations can be solved by bringing a matrix to reduced row echelon form. Learn how the elimination method corresponds to performing row operations on an augmented. The leading entry of any row is to the right. Let u be the row echelon form matrix obtained from this process. Elementary linear algebra (fourth edition), 2010. You might want to use.echelon_form() instead.
rank of a matrix using row echelon Archives Math 786
All nonzero rows are above all rows of zeros. Web learn to replace a system of linear equations by an augmented matrix. Learn how the elimination method corresponds to performing row operations on an augmented. Web to row echelon form. The leading entry of any row is to the right.
Gauss Jordan Elimination & Reduced Row Echelon Form RREF YouTube
Information and translations of row echelon form in the most comprehensive dictionary definitions resource on the web. Web to row echelon form. Web solution the correct answer is (b), since it satisfies all of the requirements for a row echelon matrix. The leading entry of any row is to the right. The leading entry of each nonzero row after the.
Row Echelon Form YouTube
Web solution the correct answer is (b), since it satisfies all of the requirements for a row echelon matrix. Web learn to replace a system of linear equations by an augmented matrix. The other matrices fall short. Below are a few examples of matrices in row echelon form: Web what does row echelon form mean?
ROW ECHELON METHOD YouTube
Web to row echelon form. A matrix is in row echelon form if it meets the following requirements: Web about press copyright contact us creators advertise developers terms privacy policy & safety how youtube works test new features press copyright contact us creators. The other matrices fall short. Web 1) 1) if you see a row which is a linear.
2.3 Reduced Row Echelon Form YouTube
Below are a few examples of matrices in row echelon form: Web what does row echelon form mean? Web about press copyright contact us creators advertise developers terms privacy policy & safety how youtube works test new features press copyright contact us creators. The leading entry of any row is to the right. Information and translations of row echelon form.
Row Echelon Form & Back Substitution YouTube
Web to row echelon form. The leading entry in row 1 of matrix a is to the right. All zero rows are at the bottom of the matrix. Web what does row echelon form mean? Let’s take an example matrix:
Linear Algebra Example Problems Reduced Row Echelon Form YouTube
You might want to use.echelon_form() instead. Web 1) 1) if you see a row which is a linear combination of the other rows then you can immediately replace it with a row of zeroes. The leading entry in row 1 of matrix a is to the right. A matrix is in row echelon form if it meets the following requirements:.
Row Echelon Form of a Matrix YouTube
The other matrices fall short. The leading entry of any row is to the right. All zero rows are at the bottom of the matrix. Elementary linear algebra (fourth edition), 2010. Web instead of gaussian elimination and back substitution, a system of equations can be solved by bringing a matrix to reduced row echelon form.
Elementary Linear Algebra Echelon Form of a Matrix, Part 1 YouTube
All nonzero rows are above all rows of zeros. Web solution the correct answer is (b), since it satisfies all of the requirements for a row echelon matrix. Below are a few examples of matrices in row echelon form: Web definition (row echelon form) a matrix m is said to be in row echelon form (ref) iff: Web to row.
linear algebra Understanding the definition of row echelon form from
The leading entry of each nonzero row after the first occurs to the right of the leading entry of the previous row. Technically, the leading coefficient can. All nonzero rows are above all rows of zeros. Let u be the row echelon form matrix obtained from this process. Web what does row echelon form mean?
The Leading Entry In Row 1 Of Matrix A Is To The Right.
Web you are using the function of sympy: Web what does row echelon form mean? All zero rows are at the bottom of the matrix. Let’s take an example matrix:
Below Are A Few Examples Of Matrices In Row Echelon Form:
You might want to use.echelon_form() instead. Web about press copyright contact us creators advertise developers terms privacy policy & safety how youtube works test new features press copyright contact us creators. ⎡⎣⎢ 3 7 −1 1 4 −2 2 2. The leading entry of any row is to the right.
Technically, The Leading Coefficient Can.
A matrix is in row echelon form if it meets the following requirements: The leading entry of each nonzero row after the first occurs to the right of the leading entry of the previous row. Information and translations of row echelon form in the most comprehensive dictionary definitions resource on the web. Let u be the row echelon form matrix obtained from this process.
Web Solution The Correct Answer Is (B), Since It Satisfies All Of The Requirements For A Row Echelon Matrix.
Web 1) 1) if you see a row which is a linear combination of the other rows then you can immediately replace it with a row of zeroes. Elementary linear algebra (fourth edition), 2010. All nonzero rows are above all rows of zeros. The other matrices fall short.